A rectangle is a flat quadrilateral with four right angles and whose parallel sides are equal to each other; if a rectangle has all four sides equal, it is called a square. The perimeter of a geometric object is the sum of the lengths of all sides. The area is instead the product of the length times the width of the figure.
Steps
Part 1 of 2: Calculate the Area

Step 1. Make sure the geometric figure is really a rectangle
The image above shows a rectangle whose horizontal sides are equal to each other, as well as the pair of vertical sides. The upper side is parallel to the lower one and the vertical ones are parallel to each other; furthermore, each horizontal side is orthogonal to each vertical side.
- If all sides are identical, you are facing a square; squares represent a class of rectangles.
- If the object you are looking at does not meet these criteria, it is not a rectangle.

Step 2. Write the formula for the area of a rectangle:
A = b x h. In this equation A indicates the area, b the length of the base of the rectangle and h its height. The unit of measurement of the surface is raised to the second power: square centimeters, square meters, square millimeters, and so on.
The units of measurement look similar: m2, cm2, mm2.
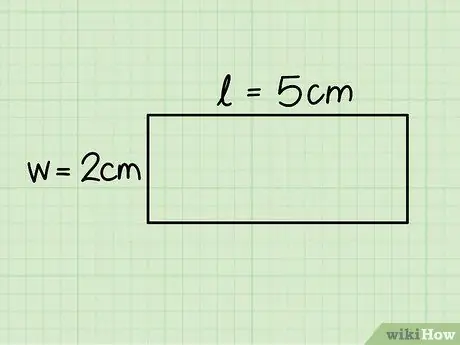
Step 3. Identify the base and height of the rectangle
The first corresponds to the length of the horizontal side, while the height is equal to the vertical side; measure both sides using a ruler to determine their length.
In the example considered, the base measures 5 cm and the height 2 cm
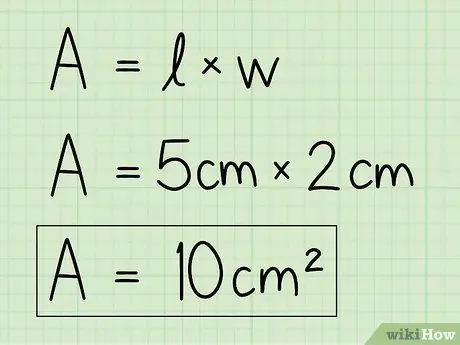
Step 4. Replace the variables with your own data to solve the equation
Use the base and height information and enter them into the formula to find the area. Multiply the base by the height.
For example, A = b x h = 5 x 2 = 10 cm2.
Part 2 of 2: Finding the Perimeter
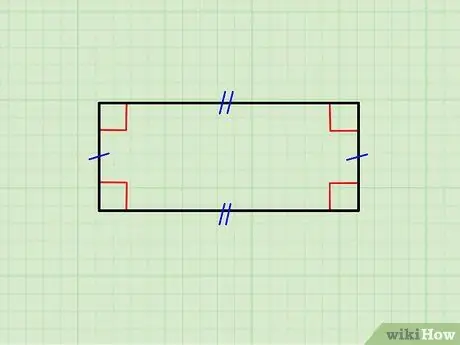
Step 1. Make sure the geometric figure is really a rectangle
The image above shows a rectangle whose horizontal sides are equal to each other, as well as the pair of vertical sides. The upper side is parallel to the lower one and the vertical ones are parallel to each other; furthermore, each horizontal side is orthogonal (forms a 90 ° angle) to each vertical side.
- If all sides are identical, you are facing a square; squares represent a class of rectangles.
- If the object you are looking at does not meet these requirements, it is not a rectangle.
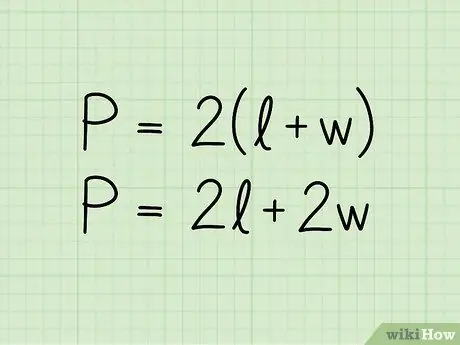
Step 2. Write the formula for the perimeter of a rectangle:
P = 2 (b + h). In the equation P represents the perimeter, b the length of the base and h that of the height. The formula could also be presented in the format P = 2b + 2h; it is the same equation written in a slightly different way.
The units of measurement of the perimeter are those of the length: centimeters, meters, millimeters, and so on

Step 3. Identify the base and height of a rectangle
The first corresponds to one of the horizontal sides and the second to one of the vertical ones; measure these dimensions with the help of a ruler.
In the previous example we considered a rectangle with a base of 5 cm and a height of 2 cm

Step 4. Replace the variables and solve the equation
Using the information you just found, solve the equation to find the perimeter; you can proceed in two ways, depending on the format in which the equation is expressed. If you are using P = 2 (b + h), add the base with the height and multiply the result by 2; if you have opted for P = 2b + 2h, double the length of the base, that of the height and add the products together.
- For example, P = 2 (b + h) = 2 (2 + 5) = 2 (7) = 14 cm.
- For example, P = 2b + 2h = (2 x 2) + (2 x 5) = 4 + 10 = 14 cm.






